Abstract
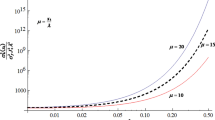
This paper investigates analytical solutions of stochastic Darcy flow in randomly heterogeneous porous media. We focus on infinite series solutions of the steady-state equations in the case of continuous porous media whose saturated log-conductivity (lnK) is a gaussian random field. The standard deviation of lnK is denoted ‘σ’. The solution method is based on a Taylor series expansion in terms of parameter σ, around the value σ=0, of the hydraulic head (H) and gradient (J). The head solution H is expressed, for any spatial dimension, as an infinite hierarchy of Green's function integrals, and the hydraulic gradient J is given by a linear first-order recursion involving a stochastic integral operator. The convergence of the ‘σ-expansion’ solution is not guaranteed a priori. In one dimension, however, we prove convergence by solving explicitly the hierarchical sequence of equations to all orders. An ‘infinite-order stochastic solution is obtained in the form of a σ-power series that converges for any finite value of σ. It is pointed out that other expansion methods based on K rather than lnK yield divergent series. The infinite-order solution depends on the integration method and the boundary conditions imposed on individual order equations. The most flexible and general method is that based on Laplacian Green's functions and boundary integrals. Imposing zero head conditions for all orders greater than one yields meaningful far-field gradient conditions. The whole approach can serve as a basis for treatment of higher-dimensional problems.
Similar content being viewed by others
References
Ababou, R.; Gelhar, L.W.; McLaughlin, D. 1988: Three-dimensional flow in random porous media, Report No. 318, Ralph Parsons Laboratory, Massachusetts Institute of Technology, Cambridge MA, 2 Vols., 833 pp
Ababou, R.; McLaughlin, D.; Gelhar, L.W.; Tompson, A.F.B. 1989: Numerical simulation of three dimensional saturated flow in randomly heterogeneous porous media, Transport in Porous Media 4, 549–565
Ababou, R.; Gelhar, L.W. Self-similar randomness and spectral conditioning: Analysis of scale effects in subsurface hydrology, Chap. XIV in Dynamic of Fluids in Hierarchical Porous Media, J. Cushman ed., Academic Press, New York NY, 505 pp
Adler, R.J. 1981: The geometry of random fields, Wiley, New York, 280pp
Adomian, G. 1980: Stochastic systems analysis, in Applied Stochastic Processes, ed. G. Adomian, Academic Press, New York, pp. 1–17
Adomian, G. 1983: Stochastic systems, Academic Press, New York
Bakr, A.A.; Gelhar, L.W.; Gutjahr, A.L.; McMillan, R.J. 1978: Stochastic analysis of spatial variability in subsurface flow, 1: Comparison of one and three dimensional flows, Water Resour. Res. 14(2)
Beran, M.J. 1968: Statistical continuum theories, Interscience Publisher (Wiley), New York, 424 pp
Bender, C.M.; Orszag, S.A. 1978: Advanced mathematical methods for scientists and engineers, McGraw-Hill, New York, 593 pp
Christakos, G. 1992: Random field models in earth sciences, Academic Press, San Diego, California
Christakos, G.; Miller, C.T.; Oliver, D. 1993: The development of stochastic space transformation and diagrammatic perturbation techniques in subsurface hydrology, Stochastic Hydrology and Hydraulics 7(1), 14–32
Dagan, G. 1985: A note on the higher-order corrections of the head covariances in steady aquifer flow, Water Resour. Res 21(4), 573–578
Dagan, G. 1993: Higher-order correction of effective permeability of heterogeneous isotropic formations of lognormal conductivity distribution, Transport in Porous Media 12, 279–290
Gelhar, L.W.; Axness, C.L. 1983: Three dimensional stochastic analysis of macrodispersion in aquifers, Water Resour. Res. 19(1), 161–180
Greenberg, M.D. 1971: Application of Green's functions in science and engineering, Prentice-Hall, Englewood Cliffs, NJ, 141 pp
Gutjhar, A.L.; Gelhar, L.W. 1981: Stochastic models for subsurface flow: Infinite versus finite domains and stationarity, Water Resour. Res. 17(2), 337–350
Isserlis, L. 1918: On a formula for the product-moment coefficient in any number of variables, Biometrica 12(1–2), 134–139
Monin, A.S.; Yaglom, A.M. 1965: Statistical fluid mechanics: Mechanics of turbulence, Volume 2, ed. J.L. Lumley, The MIT Press, Cambridge, MA, 874 pp
Neuman, S.P.; Orr, S. 1993: Prediction of steady-state flow in nonuniform geologic media by conditional moments: Exact nonlocal formalism, effective conductivities, and weak approximation, Water Resour. Res. 29(2) 341–364
Vanmarcke, E. 1983: Random fields analysis and synthesis, The MIT Press, Cambridge, MA, 382 pp
Yaglom, A.M. 1962: Stationary random functions, transl. and ed. R.A. Silverman, Dover, New York, 235 pp
Author information
Authors and Affiliations
Rights and permissions
About this article
Cite this article
Ababou, R. Solution of stochastic groundwater flow by infinite series, and convergence of the one-dimensional expansion. Stochastic Hydrol Hydraul 8, 139–155 (1994). https://doi.org/10.1007/BF01589894
Issue Date:
DOI: https://doi.org/10.1007/BF01589894