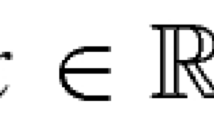Abstract
In this paper, we study the following logarithmic Schrödinger–Bopp–Podolsky system
where \(\varepsilon \) is a small positive parameter and \(V(x)\in C({\mathbb {R}}^3,{\mathbb {R}})\). Under the global condition on potential V(x), we prove the existence of positive solution \(u_{\varepsilon }\in H^1({\mathbb {R}}^3)\) of above system for \(\varepsilon >0\) small enough by applying the Variational Methods developed by Szulkin for the functional which is a sum of a \(C^1\) functional with a convex lower semicontinuous functional. Moreover, we also investigate the concentration behavior of \(\{u_{\varepsilon }\}\) as \(\varepsilon \rightarrow 0\).
Similar content being viewed by others
References
Bopp, F.: Eine Lineare Theorie des Elektrons. Ann. Phys. 430, 345–384 (1940)
Podolsky, B.: A generalized electrodynamics. Phys. Rev. 62, 68–71 (1942)
Frenkel, F.: \(4/3\) problem in classical electrodynamics. Phys. Rev. E 54, 5859–5862 (1996)
d’venia, P., Siciliano, G.: Nonlinear Schrödinger equation in the Bopp–Podolsky electrodynamics: solutions in the electrostatic case. J. Differ. Equ. 267, 1025–1065 (2019)
Bertin, M.C., Pimentel, B.M., Valccel, C.E., Zambrano, G.E.R.: Hamilton–Jacobi formalism for Podolskys electromagnetic theory on the null-plane. J. Math. Phys. 58, 082902 (2017)
Bonin, C.A., Pimentel, B.M., Ortega, P.H.: Multipole Expansion in Generalized Electrodynamics. preprint, arXiv:1608.00902
Bufalo, R., Pimentel, B.M., Soto, D.E.: Causal approach for the electron-positron scattering in generalized quantum electrodynamics. Phys. Rev. D 90, 085012 (2014)
Bufalo, R., Pimentel, B.M., Soto, D.E.: Normalizability analysis of the generalized quantum electrodynamics from the causal point of view. Int. J. Mod. Phys. A 32, 1750165 (2017)
Yang, J., Chen, H.B., Liu, S.L.: The existence of nontrivial solution of a class of Schrödinger–Bopp–Podolsky system with critical growth. Bound. Value Prob. 2020, 144 (2020)
Mascaro, B., Siciliano, G.: Positive solutions for a Schrödinger–Bopp–Podolsky system. arXiv:2009.08531v1 [math.AP] 17 Sep 2020
Gilbarg, D., Trudinger, N.S.: Elliptic Partial Differential Equations of Second Order, vol. 224, 2nd edn. Grundlehren der Mathematischen Wissenschaften. Springer, Berlin (1983)
Rabinowitz, P.H.: Minimax Methods in Critical Points Theory with Application to Differential Equations, CBMS Regional Conf. Ser. Math. vol. 65. Am. Math. Soc. Providence (1986)
Struwe, M.: Variational Methods, Applications to Nonlinear Partial Differential Equations and Hamiltonian Systems, 2nd edn. Springer, Berlin (1996)
Willem, M.: Minimax Theorems. Progress in Nonlinear Differential Equations and Their Applications. vol. 24, Birkhäuser Boston Inc., Boston (1996)
Zloshchastiev, K.G.: Logarithmic nonlinearity in the theories of quantum gravity: origin of time and observational consequences. Grav. Cosmol. 16, 288–297 (2010)
d’Avenia, P., Montefusco, E., Squassina, M.: On the logarithmic Schrödinger equation. Commun. Contemp. Math. 16, 1350032 (2014)
Degiovanni, M., Zani, S.: Multiple solutions of semilinear elliptic equations with one-sided growth conditions, nonlinear operator theory. Math. Comput. Model. 32, 1377–1393 (2000)
d’Avenia, P., Squassina, M., Zenari, M.: Fractional logarithmic Schrödinger equations. Math. Methods Appl. Sci. 38, 5207–5216 (2015)
Squassina, M., Szulkin, A.: Multiple solutions to logarithmic Schrödinger equations with periodic potential. Cal. Var. Partial Differ. Equ. 54, 585–597 (2015)
Szulkin, A.: Minimax principles for lower semicontinuous functions and applications to nonlinear boundary value problems. Ann. Inst. H. Poincaré Anal. Non Linéaire 3, 77–109 (1986)
Tanaka, K., Zhang, C.: Multi-bump solutions for logarithmic Schrödinger. Cal. Var. Partial Differ. Equ. https://doi.org/10.1007/s00526-017-1122-z
Ji, C., Szulkin, A.: A logarithmic Schrödinger equation with asymptotic conditions on the potential. J. Math. Anal. Appl. 437, 241–254 (2016)
Alves, C.O., de Morais Filho, D.C.: Existence and concentration of positive solutions for a Schrödinger logarithmic equation. Z. Angew. Math. Phys. 69, 144 (2018)
Lieb, E.H., Loss, M.: Analysis, 2nd edn. Graduate Studies in Math. 14. AMS, Providence (2001)
Lions, P.L.: The concentration-compactness principle in the calculus of variations. The locally compact case, part 2. Anal. Inst. H. Poincaré, Sect. C 1, 223–253 (1984)
Acknowledgements
This research is supported by the National Natural Science Foundation of China, Grant No. 11171220.
Author information
Authors and Affiliations
Corresponding author
Additional information
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
This research is supported by the National Natural Science Foundation of China, Grant No. 11171220.
Rights and permissions
About this article
Cite this article
Peng, X., Jia, G. Existence and concentration behavior of solutions for the logarithmic Schrödinger–Bopp–Podolsky system. Z. Angew. Math. Phys. 72, 198 (2021). https://doi.org/10.1007/s00033-021-01633-4
Received:
Revised:
Accepted:
Published:
DOI: https://doi.org/10.1007/s00033-021-01633-4
Keywords
- Logarithmic Schrödinger–Bopp–Podolsky system
- Variational methods
- Positive solutions
- Concentration behavior