Abstract
In this paper we analyze an inter-temporal optimization problem of a representative firm that invests in horizontal and vertical innovations and that faces a constraint with respect to total R&D spending. We find that there can exist two different steady-states of the economy when the amount of research spending falls short of an endogenously determined threshold: one with higher productivities and less new technologies being developed, and the other with more technologies being created and lower productivities. But, for a higher amount of R&D spending the steady-state becomes unique and the firm produces the whole spectrum of available technologies. Thus, a lock-in effect may arise that, however, can be overcome by raising R&D spending sufficiently.


Similar content being viewed by others
Notes
Or assuming revenue function to be linear in the state of quality with prices being linear functions of quality.
The budget on R&D could be set endogenously by a specific firm’s R&D policy. It could positively depend on total profits, but, this is difficult to model. Pure exogenous dynamics will not change the qualitative results: the constraint is either binding or not.
It is fairly straightforward to relax this assumption by allowing decreasing efficiency of investment in new technologies, \(\xi (n):\,\frac{\partial \xi (n)}{\partial n}<0\). Qualitative results of the paper will continue to hold, but the dynamics is more elaborated. We assume a bounded state space to keep the analysis simple and clear.
Condition (5) points to the fact that it requires efforts g(i, t) and time to develop a new technology up to the level that it becomes productive, \(q(i,t)>0\).
For an increasing \(\psi (i)\) function there would be no trade-off between expanding the range of technologies and improving the existing ones and, thus, a lock-in could not arise.
Since horizontal and vertical innovations are interrelated, it also leads to an increased complexity of invention of new products.
We do not distinguish between complex and real roots, since this affects only stability and not the existence of steady states
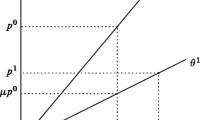
For \(R=4\), the lower \(\dot{\lambda }_n=0\) isocline starts at \(n=1,\lambda _n=0\), the upper \(\dot{\lambda }_n=0\) isocline starts at \(n=1,\lambda _n>0\). For \(R>4\), the lower \(\dot{\lambda }_n=0\) isocline also starts at a value \(\lambda _n>0\) and \(n=1\).
Formally, this is seen from (A.5) as follows: \(\dot{n}=0\) implies \(u=0\) leading to \(l=\xi \tilde{\lambda }_n\), giving \(\dot{q}=\psi _c \sqrt{1-i}\left( \frac{\psi _c \sqrt{1-i}}{r+\beta }-\xi \tilde{\lambda }_n\right) -\beta q\). Since the first term is constant, q converges to its steady-state value.
Further, there is no way to usefully spend the additional R&D on quality improvement because all invented technologies have already reached their maximal quality levels in the steady-state.
References
Acemoglu D, Aghion P, Bursztyn L, Hemous D (2012) The environment and directed technical change. Am Econ Rev 102(1):131–66
Aghion P, Howitt P (1992) A model of growth through creative destruction. Econometrica 60(2):323–351
Baveja A, Feichtinger G, Hartl R, Haunschmied J, Kort P (2000) A resource-constrained optimal control model for crackdown on illicit drug markets. J Math Anal Appl 249(1):53–79
Belyakov A, Tsachev T, Veliov V (2011) Optimal control of heterogeneous systems with endogenous domain of heterogeneity. Appl Math Optim 64(2):287–311
Bondarev A (2012) The long run dynamics of heterogeneous product and process innovations for a multi product monopolist. Econ Innov New Technol 21(8):775–799
Dawid H, Greiner A, Zou B (2010) Optimal foreign investment dynamics in the presence of technological spillovers. J Econ Dyn Control 34(3):296–313
Fattorini H (1999) Infinite dimensional optimization and control theory. Cambridge University Press, Cambridge
Hopenhayn H, Mitchell M (2001) Innovation variety and patent breadth. Rand J Econ 32(3):152–166
Lambertini L (2003) The monopolist optimal R&D portfolio. Oxf Econ Pap 55(4):561–578
Lambertini L, Orsini R (2001) Network externalities and the overprovision of quality by a monopolist. South Econ J 67(2):969–982
Lin P (2004) Process and product R&D by a multiproduct monopolist. Oxf Econ Pap 56(4):735–743
Romer P (1990) Endogenous technological change. J Polit Econ 98(5):S71–S102
Schumpeter J (1942) Capitalism, socialism and democracy. Harper & Row, New York
Seierstad A, Sydsaeter K (1999) Optimal control theory with economic applications. Elsevier, Netherlands
Skritek B, Stachev T, Veliov V (2014) Optimality conditions and the hamiltonian for a distributed optimal control problem on controlled domain. Appl Math Optim 70:141–164
van den Bergh JC (2008) Optimal diversity: increasing returns versus recombinant innovation. J Econ Behav Organ 68(3–4):565–580
Zeppini P, van den Bergh JC (2013) Optimal diversity in investments with recombinant innovation. Struct Change Econ Dyn 24(C):141–156
Author information
Authors and Affiliations
Corresponding author
Additional information
Financial support from the Bundesministerium für Bildung und Forschung (BMBF) is gratefully acknowledged (Grant 01LA1105C). This research is part of the project ‘Climate Policy and the Growth Pattern of Nations (CliPoN)’. We thank an anonymous referee for comments that helped to improve the paper.
Appendices
Appendix A: Derivation of the dynamic system
In (14a) we posit that the standard transversality conditions for the finite time horizon T also holds for the infinite time horizon, with \(\lim \nolimits _{t\rightarrow \infty }\) replacing \(\lim \nolimits _{t\rightarrow T}\). For \(\lambda _{q}\) this implies:
with \(\beta \) coming from the fundamental matrix of the quality system following Skritek et al. (2014). Then, the co-state for each technology productivity can be obtained from (13) as:
which yields a constant shadow price in time for each technology for the infinite horizon case, i.e. for \(T\rightarrow \infty \),
The form of l(t) results from substituting (9), (10) into (11) taking into account specification (7) and the form of the co-state variable \(\lambda _{q}(i,t)={1}/({r+\beta })\):
which is a function of the variety expansion and of its co-state variable. The differential equations for all system variables, then, are obtained by substituting the controls in (9), (10) with l(t) defined in (A.4). The resulting productivities, q(i, t), are functions of the variety of technologies, n(t), and of the resource constraint R which enters optimal investments through the Lagrange multiplier:
Using the expressions for the controls, (9), (10), the Lagrange multiplier, (A.4), and the efficiency of investments into the boundary technology, \(\psi (n)=\psi _c \sqrt{1-n(t)}\), one can obtain the explicit expression for the change of the co-state variable as a function of n(t) and of the budget constraint R:
Substitution of (9) with l(t), given by (A.4), into the dynamics of variety expansion from (2), yields the following differential equation for n(t):
Appendix B: Proof of proposition 3
To prove the first part, we first note that the inital value of n, \(n(0)=n_0<1\), is given whereas the initial value for \(\lambda \), \(\lambda (0)\), can be chosen freely by the optimizing firm. Then, we see from Fig. 1a that starting above the lower \(\dot{\lambda }_n=0\) isocline implies that n(t) rises and \(\lambda (t)\) rises (declines) if the initial \(\lambda (0)\) is chosen below (above) the upper \(\dot{\lambda }_n=0\) isocline. Since n(t) cannot decline, limit cycles are excluded. Therefore, and since this is a two dimensional system in the plane, the only feasible solution in that case is convergence to the steady-state \((\tilde{n}=1,\tilde{\lambda }_n>0)\).
In the case of two steady-states, the eigenvalues of the Jacobian matrix evaluated at the steady-state are symmetric around r / 2. For the parameter values in Table 1, the steady-state with the lower \(\tilde{n}\) is a saddle point, with the eigenvalues given by 0.9424 and \(-0.8924\) and the steady-state with the higher \(\tilde{n}\) is an unstable focus, with the eigenvalues given by \(0.025\pm 1.02576\, i\), where \(i=\sqrt{-1}\). The same qualitative result is obtained for \(R=0.1\) and all other parameter values unchanged. Qualitatively, the result also remains unchanged when setting \(r = 0.05, \psi _c = 1, \xi = 1, \beta = 0.1, R = 0.9\) and for \(r = 0.02, \psi _c = 1, \xi = 1, \beta = 0.1, R = 2.5\).
Rights and permissions
About this article
Cite this article
Bondarev, A., Greiner, A. Technology lock-in with horizontal and vertical innovations through limited R&D spending. 4OR-Q J Oper Res 16, 51–65 (2018). https://doi.org/10.1007/s10288-017-0348-0
Received:
Revised:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s10288-017-0348-0