Hostname: page-component-848d4c4894-hfldf Total loading time: 0 Render date: 2024-05-21T05:20:30.790Z Has data issue: false hasContentIssue false
  • English
  • Français

Onset of fully compressible convection in a rapidly rotating spherical shell

Published online by Cambridge University Press:  01 July 2019

Shuang Liu ,
Zhen-Hua Wan Open the ORCID record for Zhen-Hua Wan [Opens in a new window] ,
Rui Yan ,
Chao Sun Open the ORCID record for Chao Sun [Opens in a new window]  and
De-Jun Sun
Shuang Liu
Affiliation:
Department of Modern Mechanics, University of Science and Technology of China, Hefei, Anhui 230027, China
Zhen-Hua Wan*
Affiliation:
Department of Modern Mechanics, University of Science and Technology of China, Hefei, Anhui 230027, China
Rui Yan
Affiliation:
Department of Modern Mechanics, University of Science and Technology of China, Hefei, Anhui 230027, China
Chao Sun
Affiliation:
Center for Combustion Energy, Key Laboratory for Thermal Science and Power Engineering of Ministry of Education, Department of Energy and Power Engineering, Tsinghua University, Beijing 100084, China
De-Jun Sun*
Affiliation:
Department of Modern Mechanics, University of Science and Technology of China, Hefei, Anhui 230027, China
*
†Email addresses for correspondence: wanzh@ustc.edu.cn, dsun@ustc.edu.cn
†Email addresses for correspondence: wanzh@ustc.edu.cn, dsun@ustc.edu.cn
Get access Rights & Permissions [Opens in a new window]

Abstract

The onset of thermal convection in a rapidly rotating spherical shell is studied by linear stability analysis based on the fully compressible Navier–Stokes equations. Compressibility is quantified by the number of density scale heights 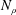$N_{\unicode[STIX]{x1D70C}}$, which measures the intensity of density stratification of the motionless, polytropic base state. The nearly adiabatic flow with polytropic index 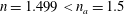$n=1.499<n_{a}=1.5$ is considered, where 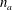$n_{a}$ is the adiabatic polytropic index. By investigating the stability of the base state with respect to the disturbance of specified wavenumber, the instability process is found to be sensitive to the Prandtl number 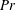$Pr$ and to 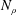$N_{\unicode[STIX]{x1D70C}}$. For large 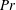$Pr$ and small 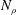$N_{\unicode[STIX]{x1D70C}}$, the quasi-geostrophic columnar mode loses stability first; while for relatively small $Pr$ a new quasi-geostrophic compressible mode is identified, which becomes unstable first under strong density stratification. The inertial mode can also occur first for relatively small $Pr$ and a certain intensity of density stratification in the parameter range considered. Although the Rayleigh numbers 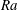$Ra$ for the onsets of the quasi-geostrophic compressible mode and columnar mode are different by several orders of magnitude, we find that they follow very similar scaling laws with the Taylor number. The critical 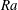$Ra$ for convection onset is found to be always positive, in contrast with previous results based on the widely used anelastic model that convection can occur at negative 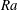$Ra$. By evaluating the relative magnitude of the time derivative of density perturbation in the continuity equation, we show that the anelastic approximation in the present system cannot be applied in the small-$Ra$ and large-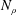$N_{\unicode[STIX]{x1D70C}}$ regime.

JFM classification

Convection: Buoyancy-driven instability Geophysical and Geological Flows: Rotating flows
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 873 , 25 August 2019 , pp. 1090 - 1115
Check for updates
Copyright
© 2019 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Batchelor, G. K. 1953 The conditions for dynamical similarity of motions of a frictionless perfect-gas atmosphere. Q. J. R. Meteorol. Soc. 79 (340), 224–235.Google Scholar
Berkoff, N. A., Kersale, E. & Tobias, S. M. 2010 Comparison of the anelastic approximation with fully compressible equations for linear magnetoconvection and magnetic buoyancy. Geophys. Astrophys. Fluid Dyn. 104 (5–6), 545–563.Google Scholar
Braginsky, S. I. & Roberts, P. H. 1995 Equations governing convection in Earth’s core and the geodynamo. Geophys. Astrophys. Fluid Dyn. 79 (1–4), 1–97.Google Scholar
Brown, B. P., Vasil, G. M. & Zweibel, E. G. 2012 Energy conservation and gravity waves in sound-proof treatments of stellar interiors. Part I. Anelastic approximations. Astrophys. J. 756 (2), 109.10.1088/0004-637X/756/2/109Google Scholar
Busse, F. H. 1970 Thermal instabilities in rapidly rotating systems. J. Fluid Mech. 44 (3), 441–460.Google Scholar
Busse, F. H. & Hood, L. L. 1982 Differential rotation driven by convection in a rapidly rotating annulus. Geophys. Astrophys. Fluid Dyn. 21 (1–2), 59–74.10.1080/03091928208209005Google Scholar
Busse, F. H. & Simitev, R. 2004 Inertial convection in rotating fluid spheres. J. Fluid Mech. 498, 23–30.10.1017/S0022112003006943Google Scholar
Busse, F. H., Zhang, K. & Liao, X. 2005 On slow inertial waves in the solar convection zone. Astrophys. J. Lett. 631 (2), L171–L174.10.1086/497300Google Scholar
Cai, T., Chan, K. L. & Deng, L. 2011 Numerical simulation of core convection by a multi-layer semi-implicit spherical spectral method. J. Comput. Phys. 230 (24), 8698–8712.Google Scholar
Calkins, M. A., Julien, K. & Marti, P. 2015a The breakdown of the anelastic approximation in rotating compressible convection: implications for astrophysical systems. Proc. R. Soc. Lond. A 471 (2175), 20140689.Google Scholar
Calkins, M. A., Julien, K. & Marti, P. 2015b Onset of rotating and non-rotating convection in compressible and anelastic ideal gases. Geophys. Astrophys. Fluid Dyn. 109 (4), 422–449.10.1080/03091929.2014.987670Google Scholar
Chan, K. L., Mayr, H. G., Mengel, J. G. & Harris, I. 1994 A ‘stratified’ spectral model for stable and convective atmospheres. J. Comput. Phys. 113 (2), 165–176.10.1006/jcph.1994.1128Google Scholar
Chandrasekhar, S. 1961 Hydrodynamic and Hydromagnetic Stability. Clarendon Press.Google Scholar
Chenoweth, D. R. & Paolucci, S. 1986 Natural convection in an enclosed vertical air layer with large horizontal temperature differences. J. Fluid Mech. 169, 173–210.Google Scholar
Dormy, E., Soward, A. M., Jones, C. A., Jault, D. & Cardin, P. 2004 The onset of thermal convection in rotating spherical shells. J. Fluid Mech. 501, 43–70.10.1017/S0022112003007316Google Scholar
Drew, S. J., Jones, C. A. & Zhang, K. 1995 Onset of convection in a rapidly rotating compressible fluid spherical shell. Geophys. Astrophys. Fluid Dyn. 80 (3–4), 241–254.10.1080/03091929508228957Google Scholar
Durran, D. R. 2008 A physically motivated approach for filtering acoustic waves from the equations governing compressible stratified flow. J. Fluid Mech. 601, 365–379.Google Scholar
Gilman, P. A. & Glatzmaier, G. A. 1981 Compressible convection in a rotating spherical shell. I. Anelastic equations. Astrophys. J. Suppl. 45, 335–349.10.1086/190714Google Scholar
Glatzmaier, G. A. & Gilman, P. A. 1981a Compressible convection in a rotating spherical shell. II. A linear anelastic model. Astrophys. J. Suppl. 45, 351–380.Google Scholar
Glatzmaier, G. A. & Gilman, P. A. 1981b Compressible convection in a rotating spherical shell. IV. Effects of viscosity, conductivity, boundary conditions, and zone depth. Astrophys. J. Suppl. 47, 103–115.10.1086/190753Google Scholar
Guillot, T. 1999a A comparison of the interiors of Jupiter and Saturn. Planet. Space Sci. 47 (10–11), 1183–1200.Google Scholar
Guillot, T. 1999b Interiors of giant planets inside and outside the solar system. Science 286 (5437), 72–77.Google Scholar
Jones, C. A., Boronski, P., Brun, A. S., Glatzmaier, G. A., Gastine, T., Miesch, M. S. & Wicht, J. 2011 Anelastic convection-driven dynamo benchmarks. Icarus 216 (1), 120–135.10.1016/j.icarus.2011.08.014Google Scholar
Jones, C. A., Kuzanyan, K. M. & Mitchell, R. H. 2009 Linear theory of compressible convection in rapidly rotating spherical shells, using the anelastic approximation. J. Fluid Mech. 634, 291–319.Google Scholar
Jones, C. A., Roberts, P. H. & Galloway, D. J. 1990 Compressible convection in the presence of rotation and a magnetic field. Geophys. Astrophys. Fluid Dyn. 53 (3), 145–182.Google Scholar
Jones, C. A., Rotvig, J. & Abdulrahman, A. 2003 Multiple jets and zonal flow on Jupiter. Geophys. Res. Lett. 30 (14), 1731.10.1029/2003GL016980Google Scholar
Jones, C. A., Soward, A. M. & Mussa, A. I. 2000 The onset of thermal convection in a rapidly rotating sphere. J. Fluid Mech. 405, 157–179.Google Scholar
Lecoanet, D., Brown, B. P., Zweibel, E. G., Burns, K. J., Oishi, J. S. & Vasil, G. M. 2014 Conduction in low Mach number flows. I. Linear and weakly nonlinear regimes. Astrophys. J. 797 (2), 94.Google Scholar
Lund, T. S. & Fritts, D. C. 2012 Numerical simulation of gravity wave breaking in the lower thermosphere. J. Geophys. Res. 117 (D21), D21105.Google Scholar
Ogura, Y. & Phillips, N. A. 1962 Scale analysis of deep and shallow convection in the atmosphere. J. Atmos. Sci. 19 (2), 173–179.Google Scholar
Proudman, J. 1916 On the motion of solids in a liquid possessing vorticity. Proc. R. Soc. Lond. A 92 (642), 408–424.Google Scholar
Roberts, P. H. 1968 On the thermal instability of a rotating-fluid sphere containing heat sources. Phil. Trans. R. Soc. Lond. A 263 (1136), 93–117.Google Scholar
Rotvig, J. & Jones, C. A. 2006 Multiple jets and bursting in the rapidly rotating convecting two-dimensional annulus model with nearly plane-parallel boundaries. J. Fluid Mech. 567, 117–140.Google Scholar
Sánchez, J., Garcia, F. & Net, M. 2016a Critical torsional modes of convection in rotating fluid spheres at high Taylor numbers. J. Fluid Mech. 791, R1.Google Scholar
Sánchez, J., García, F. & Net, M. 2016b Radial collocation methods for the onset of convection in rotating spheres. J. Comput. Phys. 308, 273–288.Google Scholar
Spiegel, E. A. & Veronis, G. 1960 On the Boussinesq approximation for a compressible fluid. Astrophys. J. 131, 442–447.Google Scholar
Takehiro, S. 2008 Physical interpretation of spiralling-columnar convection in a rapidly rotating annulus with radial propagation properties of Rossby waves. J. Fluid Mech. 614, 67–86.Google Scholar
Takehiro, S. 2010 Kinetic energy budget analysis of spiraling columnar critical convection in a rapidly rotating spherical shell. Fluid Dyn. Res. 42 (5), 055501.10.1088/0169-5983/42/5/055501Google Scholar
Taylor, G. I. 1921 Experiments with rotating fluids. Proc. R. Soc. Lond. A 100 (703), 114–121.Google Scholar
Trefethen, L. N. 2000 Spectral Methods in MATLAB. SIAM.Google Scholar
Verhoeven, J. & Glatzmaier, G. A. 2018 Validity of sound-proof approaches in rapidly-rotating compressible convection: marginal stability versus turbulence. Geophys. Astrophys. Fluid Dyn. 112 (1), 36–61.Google Scholar
Verhoeven, J., Wiesehöfer, T. & Stellmach, S. 2015 Anelastic versus fully compressible turbulent Rayleigh–Bénard convection. Astrophys. J. 805 (1), 62.Google Scholar
Yano, J.-I. 1992 Asymptotic theory of thermal convection in rapidly rotating systems. J. Fluid Mech. 243, 103–131.Google Scholar
Zhang, K. 1992 Spiralling columnar convection in rapidly rotating spherical fluid shells. J. Fluid Mech. 236, 535–556.Google Scholar
Zhang, K. 1994 On coupling between the Poincaré equation and the heat equation. J. Fluid Mech. 268, 211–229.Google Scholar
Zhang, K. 1995 On coupling between the Poincaré equation and the heat equation: non-slip boundary condition. J. Fluid Mech. 284, 239–256.Google Scholar
Zhang, K. & Busse, F. H. 1987 On the onset of convection in rotating spherical shells. Geophys. Astrophys. Fluid Dyn. 39 (3), 119–147.Google Scholar
Zhang, K., Lam, K. & Kong, D. 2017 Asymptotic theory for torsional convection in rotating fluid spheres. J. Fluid Mech. 813, R2.Google Scholar
Zhang, K. & Liao, X. 2017 Theory and Modeling of Rotating Fluids: Convection, Inertial Waves and Precession. Cambridge University Press.Google Scholar