Abstract
We propose a compact scheme for high-density and high-energy positron generation. In the scheme, the generation, compression and acceleration of positrons are encompassed in a plasma channel irradiated by an ultra intense laser. In about 20 μm, positrons are accelerated with their energy beyond 20 GeV, and are compressed with a maximal density of 4.1nc. The obtained positron beam with a wave-like density profile is accelerated via the direct laser acceleration.
Export citation and abstract BibTeX RIS
1. Introduction
Positron ever since its discovery in 1930s [1] has found diverse applications in science, medicine and engineering [2, 3]. For example, positrons with energies from eVs to TeVs are of great use to study the physical world. For energies from eV to hundreds of keV, they are required in material science for defect and phase transition research [4, 5]. With energies of tens of GeV, they provide a complementary tool to observe the structure of the nucleon [6]. GeVs to TeVs positron beams are used to study energetic phenomena in laboratory astrophysics [7], and to realize a lepton collider for particle physics to search for physics beyond the Standard Model [8].
To acquire high-energy, positrons are usually accelerated via a secondary accelerator. For example, in the scheme of a hollow plasma channel with quadrupole magnets [9], the acceleration field is driven by an injected proton beam. However, the acceleration gradient in that scheme is not high, which is only TeV km−1. Besides, the above acceleration scheme is complicated, and positron generation and injection are not stable. Laser induced positron generation has unique properties, such as high yield, high-density, and high-energy [10]. Positron generation via sending a high-energy electron beam into a secondary high Z converter includes the trident process and the Bethe–Heitler (BH) process [11]. In high Z converter, the positron beam generation is separated from their acceleration and compression. Compared to the trident process and the BH process [12], the multi-photon Breit–Wheeler (BW) process is an all-optical way, in which the radiated photons interact with laser photons [13]. Provided that the generated pairs further radiate photons with energies high enough, a cascade may be initiated [14]. In the BW process, the radiation processes are varied with different setups of the target. As a thin (1 μm) solid target is used, photons are radiated via the collision of incident laser with electrons provided by the reflected wave [15, 16]. In uniform plasmas, electrons in front of the laser self-inject into the pulse to maintain the balance between the ponderomotive potential and the electrostatic potential [17]. When two counter-propagating pulses irradiate a cylinder channel, electrons are extracted by the transverse electric field [18]. Two counter-propagating pulses schemes are favorable to enhance the positron generation, but unfavorable to the positron acceleration in one direction [8].
In this paper, we propose a compact scheme encompassing the generation, compression and acceleration of high-flux positrons. Two different radiation processes are considered. When an ultra intense laser with the dimensionless laser parameter a = 900 (2.2 × 1024 W cm−2, 140 PW) irradiates a plasma channel, 1.535 × 1010 positrons are generated via the BW process. Due to the strong electromagnetic field generated in the channel, positrons are accelerated with a cutoff energy of >20 GeV in ∼20 μm, and compressed with a maximal density of 4.1nc. Mechanisms of positron generation and acceleration, as well as the laser self-focusing, are discussed in detail. This paper is organized as below. In section 2 is the positron generation process described by both 3D and 2D simulations. Then we track the positrons and show their acceleration in section 3. The influence of laser intensity and plasma density are discussed in section 4. Finally, conclusions are presented in section 5.
2. Positron generation
The scheme is demonstrated via the relativistic particle-in-cell code EPOCH, in which the quantum electrodynamics module is implanted [19]. For clear configurations, we carry out full 3D simulations firstly. The simulation box is located in 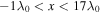 ,
, 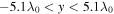 and
and 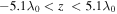 , where λ0 = 1 μm is the laser wave length. This box is divided into 600 × 340 × 340 cells with 60 macro electrons in each cell. To make the scheme more feasible in laboratory, fully ionized carbon plasmas are employed and initially set in x > 0 and r ≤ 5λ0, where
, where λ0 = 1 μm is the laser wave length. This box is divided into 600 × 340 × 340 cells with 60 macro electrons in each cell. To make the scheme more feasible in laboratory, fully ionized carbon plasmas are employed and initially set in x > 0 and r ≤ 5λ0, where 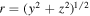 . The plasmas distribute uniformly in the x axial direction. In the radial direction, the electron density is
. The plasmas distribute uniformly in the x axial direction. In the radial direction, the electron density is 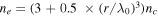 , where
, where 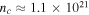 cm−3 is the critical density according to the incident laser. Thus the near-critical-density plasmas around the axis can increase the laser energy conversion efficiency [20], and the high-density plasmas outside can induce the laser self-focusing. A circularly polarized Gaussian laser pulse with a spot radius of 2λ0 is incident from the center of the left boundary, and propagates along the x axial direction. The pulse duration is 15 fs. Both the radius and the pulse duration are given in the form of the full width at half maximum (FWHM). Comparing to the linearly polarized laser, firstly, the
cm−3 is the critical density according to the incident laser. Thus the near-critical-density plasmas around the axis can increase the laser energy conversion efficiency [20], and the high-density plasmas outside can induce the laser self-focusing. A circularly polarized Gaussian laser pulse with a spot radius of 2λ0 is incident from the center of the left boundary, and propagates along the x axial direction. The pulse duration is 15 fs. Both the radius and the pulse duration are given in the form of the full width at half maximum (FWHM). Comparing to the linearly polarized laser, firstly, the 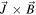 heating to the target is absent theoretically using the circularly polarized laser. Secondly, electrons/positrons will rotate around the laser axis in the circularly polarized laser, which will be in favor of trapping and acceleration. A normalized laser amplitude a = 700 is employed, corresponding to the laser peak intensity of
heating to the target is absent theoretically using the circularly polarized laser. Secondly, electrons/positrons will rotate around the laser axis in the circularly polarized laser, which will be in favor of trapping and acceleration. A normalized laser amplitude a = 700 is employed, corresponding to the laser peak intensity of 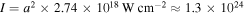 W cm−2 and the power of 86 PW.
W cm−2 and the power of 86 PW.
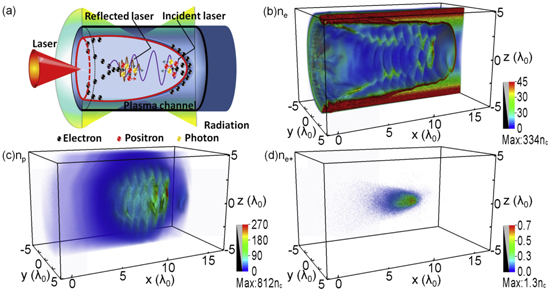
When an ultra intense laser pulse irradiates the plasma, it will interact with a single electron, and the Lorentz factor reached by an electron is  [16]. The plasma becomes relativistically transparent though it is initially overdense [21, 22]. The laser pulse expels electrons aside, leaving an electron-absent channel behind as schematically shown in figure 1(a). On the one hand, a high-density electron spike is formed in front of the laser pulse as can be seen in figure 1(b). Given by the Poisson's equation, a charge separation field is initiated as
[16]. The plasma becomes relativistically transparent though it is initially overdense [21, 22]. The laser pulse expels electrons aside, leaving an electron-absent channel behind as schematically shown in figure 1(a). On the one hand, a high-density electron spike is formed in front of the laser pulse as can be seen in figure 1(b). Given by the Poisson's equation, a charge separation field is initiated as 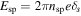 , where nsp is the density of the electron spike, and δs is the FWHM of the density peak. As the electron density increases, the field Esp gets stronger. When the charge separation field is higher than the effective field due to the laser ponderomotive force (Vp), electrons in front of the laser can overcome the pulse front and propagate against the laser [17]. Thus the electric field (
, where nsp is the density of the electron spike, and δs is the FWHM of the density peak. As the electron density increases, the field Esp gets stronger. When the charge separation field is higher than the effective field due to the laser ponderomotive force (Vp), electrons in front of the laser can overcome the pulse front and propagate against the laser [17]. Thus the electric field (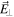 ) perpendicular to the electron velocity (
) perpendicular to the electron velocity (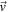 ) is superposed to the magnetic term
) is superposed to the magnetic term  , inducing the increase of the radiation controlling parameter
, inducing the increase of the radiation controlling parameter 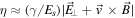 [15]. Here,
[15]. Here, 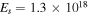 Vm−1 is the Schwinger field [23]. Radiation via the electrons Compton back-scattering in the laser field is enhanced significantly. On the other hand, due to the strong charge separation field, electrons run backwards along the channel inner wall. They are injected into the channel from the tail of the laser. It is noted from figure 1(b) that when injected from the tail, electrons are accelerated in the laser field, accompanied with strong oscillation transversely. The electron oscillation also contributes to photon radiations. Both radiation processes will be in favor of the BW positron generation. At t = 18T0 (T0 ≈ 3.3 fs), the maximal density of radiated photons is far beyond 270nc (figure 1(c)). These high-density photons further interact with the laser photons to generate copious positrons. It is noted from figure 1(d) that the positron maximal density is already ∼1.3nc at t = 18T0. At a later time, the positron number and density become even higher. In addition, considering the initial electron density distribution (dense outside and rare inside), the laser pulse is confined in the channel with the laser self-focusing being induced.
Vm−1 is the Schwinger field [23]. Radiation via the electrons Compton back-scattering in the laser field is enhanced significantly. On the other hand, due to the strong charge separation field, electrons run backwards along the channel inner wall. They are injected into the channel from the tail of the laser. It is noted from figure 1(b) that when injected from the tail, electrons are accelerated in the laser field, accompanied with strong oscillation transversely. The electron oscillation also contributes to photon radiations. Both radiation processes will be in favor of the BW positron generation. At t = 18T0 (T0 ≈ 3.3 fs), the maximal density of radiated photons is far beyond 270nc (figure 1(c)). These high-density photons further interact with the laser photons to generate copious positrons. It is noted from figure 1(d) that the positron maximal density is already ∼1.3nc at t = 18T0. At a later time, the positron number and density become even higher. In addition, considering the initial electron density distribution (dense outside and rare inside), the laser pulse is confined in the channel with the laser self-focusing being induced.
Figure 1. Schematic of an ultra intense laser irradiating a plasma channel (a). Density distributions of electrons (b), photons (c) and positrons (d) in unit of nc at t = 18T0 in 3D simulation with a = 700.
Download figure:
Standard image High-resolution imageLimited by the computation source and the data size, we also conducted 2D simulations with higher resolutions to explore the underlying physics in detail. The cell size is decreased to 0.0075λ0, which is comparable to the Debye length of the plasma [24]. Besides, a moving window is opened in the x axial direction. The window starts to move at t = 17T0 with a speed of vx = 0.93c, where c is the light speed in vacuum. In 2D simulations, two normalized laser amplitudes a = 700 and 900 are employed. Actually, when a = 70 (i.e. ∼1.3 × 1022 W cm−2), an intensity with state-of-art laser facilities, positrons generation and acceleration can be still realized in the channel. Here, for detailed investigations, higher intensity lasers are employed to generate more positrons with clearer density profiles.
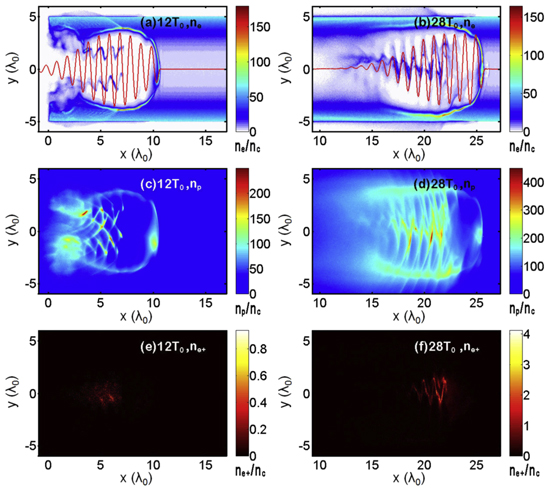
In 2D case, clearer simulation results are provided. Figures 2(a) and (b) show the density distributions of electrons at t = 12T0 and 28T0. It is noted from figure 2(a) that a bubble-like channel is formed at t = 12T0. An electron spike with density of ∼100nc is accumulated in front of the laser pulse, while two electron beams oscillating in the laser field with the wave length of λ ≈ λ0. The oscillating electrons experience the Direct Laser Acceleration (DLA) [25]. When an electron radiates photons, it will suffer a radiation reaction force (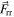 ), which can be expressed as
), which can be expressed as ![${\vec{F}}_{{\rm{rr}}}\,\approx -(2{e}^{4}/3{m}_{e}^{2}{c}^{5}){\gamma }^{2}\vec{v}[{\left(\vec{E}+\vec{v}\times \vec{B}/c\right)}^{2}-{\left(\vec{E}\cdot \vec{v}\right)}^{2}/{c}^{2}]$](https://content.cld.iop.org/journals/0741-3335/61/6/065014/revision2/ppcfab07f3ieqn17.gif) [26]. Electrons are recoiled back into the laser field (figure 2(b)) which is known as the Radiation Reaction Trapping (RRT). The RRT is favorable in electrons acceleration, and thus further radiation and positron generation. Photon radiation in the bubble mainly results from the oscillation of electrons as noted from the photon density distribution in figures 2(c) and (d). Photons at x = 10λ0 in figure 2(c) are mainly contributed by the Compton back-scattering process. The maximal photon density is around 220nc at t = 12T0. As the laser propagates deeply in the plasma channel, more photons are radiated. At t = 28T0, the maximal photon density is ∼440nc, twice of that at t = 12T0. Firstly, it is due to more electrons injected and higher energy electron acquired. Secondly, the RRT also enhances the radiation. The photon energy density is ∼6.503 × 1018 Jm−3 at t = 28T0, seven orders of magnitude higher than the high-energy-density threshold (1011 Jm−3) [27]. These high-density and high-energy-density photons are in favor of the multi-photon BW process. It can be seen in figure 2(e) that copious positrons have already been generated with a density of ∼nc at t = 12T0. These positrons mainly originate from the region of high-density photons. At t = 28T0, more positrons (1.535 × 1010) are generated with a higher density (4.1nc). It is noted in figure 2(f) that these positrons are evolved with a wave-like density profile (
[26]. Electrons are recoiled back into the laser field (figure 2(b)) which is known as the Radiation Reaction Trapping (RRT). The RRT is favorable in electrons acceleration, and thus further radiation and positron generation. Photon radiation in the bubble mainly results from the oscillation of electrons as noted from the photon density distribution in figures 2(c) and (d). Photons at x = 10λ0 in figure 2(c) are mainly contributed by the Compton back-scattering process. The maximal photon density is around 220nc at t = 12T0. As the laser propagates deeply in the plasma channel, more photons are radiated. At t = 28T0, the maximal photon density is ∼440nc, twice of that at t = 12T0. Firstly, it is due to more electrons injected and higher energy electron acquired. Secondly, the RRT also enhances the radiation. The photon energy density is ∼6.503 × 1018 Jm−3 at t = 28T0, seven orders of magnitude higher than the high-energy-density threshold (1011 Jm−3) [27]. These high-density and high-energy-density photons are in favor of the multi-photon BW process. It can be seen in figure 2(e) that copious positrons have already been generated with a density of ∼nc at t = 12T0. These positrons mainly originate from the region of high-density photons. At t = 28T0, more positrons (1.535 × 1010) are generated with a higher density (4.1nc). It is noted in figure 2(f) that these positrons are evolved with a wave-like density profile (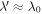 ). As soon as generated in the laser field, positrons are compressed to a higher density while being accelerated via the DLA. By estimating, ∼8.05% of the laser energy is converted into γ-photons, and ∼0.079% into positrons. The laser energy conversion efficiency to positrons is much higher than that in the positron generation BH process (∼0.02%) [28].
). As soon as generated in the laser field, positrons are compressed to a higher density while being accelerated via the DLA. By estimating, ∼8.05% of the laser energy is converted into γ-photons, and ∼0.079% into positrons. The laser energy conversion efficiency to positrons is much higher than that in the positron generation BH process (∼0.02%) [28].
Figure 2. Density distributions of electrons ((a) and (b)), photons ((c) and (d)) and positrons ((e) and (f)) in unit of nc at t = 12T0 ((a), (c) and (e)) and 28T0 ((b), (d) and (f)) in 2D simulation with a = 900. The profiles of laser electric field Ey along the x axis are also presented in (a) and (b).
Download figure:
Standard image High-resolution image3. Positron acceleration
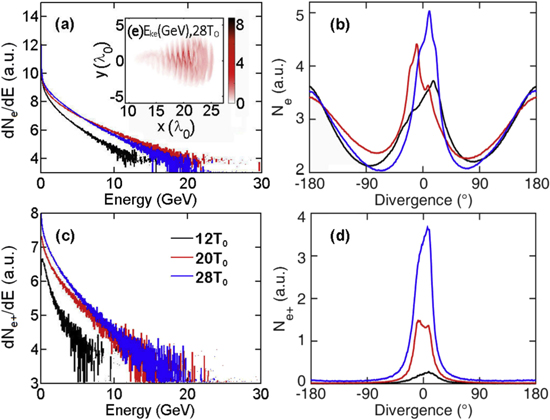
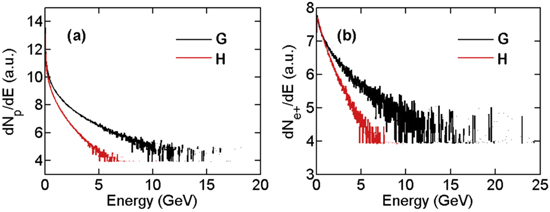
Figure 3 shows the spectrum and the divergence of electrons (positrons) in 2D simulations. Here, all electrons (positrons) in the simulation box are calculated. However, the high-energy part in the spectra only respects electrons in the bubble, as the high-energy-density electrons contributing to radiation are all in the laser pulse (figure 3(e)). Due to the DLA of electrons in the bubble and the ponderomotive force acceleration of electrons in front of the laser, the electron cutoff energy is ∼30 GeV at t = 20T0. High-energy electrons are favorable in photon emission. However, the electron cutoff energy decreases at a later time (i.e. t = 28T0). On the one hand, the electron effective temperature is  in the DLA, where α ≈ 1.5 MeV is a constant [29]. Teff is limited by the laser peak intensity. On the other hand, more electron energy are converted into photon emission. It is noted from figure 3(b) that a lot of electrons are accelerated in the x axial direction. Due to the transverse oscillation, the direction of electrons oscillates around the x axis. At the same time, a lot of electrons move backwards along the bubble inner wall as a result of the strong charge separation field. Under the DLA, the positron cutoff energy is >20 GeV with an average positron energy of ∼933 MeV at t = 28T0 (figure 3(c)). The acceleration gradient is ∼GeV μm−1. Varying around the x axis, these high-energy positrons are confined in a cone (±30°) with an average divergence angle of 25.86° (figure 3(d)). The plasma channel provides an effective trap for positron collimation [9].
in the DLA, where α ≈ 1.5 MeV is a constant [29]. Teff is limited by the laser peak intensity. On the other hand, more electron energy are converted into photon emission. It is noted from figure 3(b) that a lot of electrons are accelerated in the x axial direction. Due to the transverse oscillation, the direction of electrons oscillates around the x axis. At the same time, a lot of electrons move backwards along the bubble inner wall as a result of the strong charge separation field. Under the DLA, the positron cutoff energy is >20 GeV with an average positron energy of ∼933 MeV at t = 28T0 (figure 3(c)). The acceleration gradient is ∼GeV μm−1. Varying around the x axis, these high-energy positrons are confined in a cone (±30°) with an average divergence angle of 25.86° (figure 3(d)). The plasma channel provides an effective trap for positron collimation [9].
Figure 3. Spectrum ((a) and (c)) and divergence ((b) and (d)) of electrons ((a) and (b)) and positrons ((c) and (d)) in 2D simulation with a = 900. The kinetic energy distribution of electrons (e) at t = 28T0 is inset in (a).
Download figure:
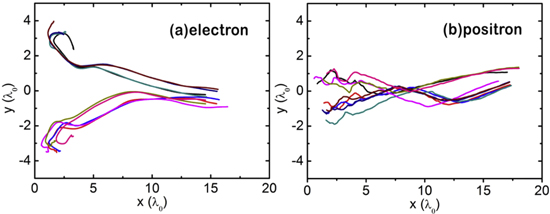
Standard image High-resolution imageTo have a clear picture of the particle motion, figure 4 presents typical electron and positron trajectories. In the following works, a normalized laser amplitude of a = 700 is employed to save computation source for massive and high resolution simulations. Noted from the electron trajectories, it is confirmed that electrons move backwards along the bubble inner wall, and then they are injected into the bubble from the tail. Two beams merge into one at the x axis while propagating in the x axial direction. It is benefit to the γ-photon radiation as the laser field around the axis is stronger. Uniformly generated in the bubble, positrons are also centralized around the x axis while being accelerated. This also agrees well with the density distribution of positrons as shown in figure 2(f).
Figure 4. Typical electron (a) and positron (b) trajectories in 2D simulation with a = 700.
Download figure:
Standard image High-resolution image4. Discussions
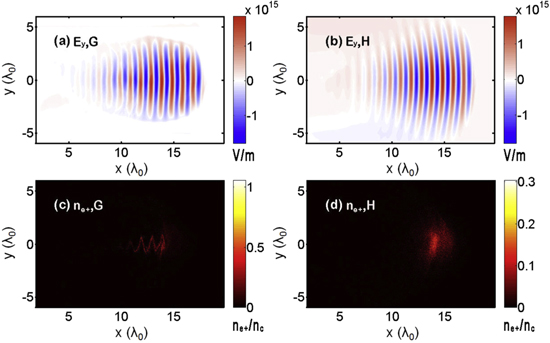
In order to investigate the influence of the transverse density gradient, it is compared to the homogeneous density distribution. In the following, G case and H case are used for short to represent the transverse Gradient density distribution case and the Homogeneous density distribution case, respectively. The electron density in the H case is 3nc. Figure 5 shows distributions of the laser transverse electric field and the positron density at t = 20T0 in two cases. When an ultra intense laser irradiates the plasma channel, a bubble-like channel is formed. Due to the surrounding high-density plasmas, the laser pulse is confined in the bubble, and the laser self-focusing is initiated to increase its intensity. To realize the laser self-focusing, the regarded condition should be met: 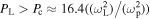 GW [30], where PL is the laser power, Pc is the critical power for laser self-focusing, and ωL and ωp are frequencies of the laser and the plasma, respectively. This condition is satisfied in the G case. According to the electric field in both cases, the ratio between laser peak intensities is
GW [30], where PL is the laser power, Pc is the critical power for laser self-focusing, and ωL and ωp are frequencies of the laser and the plasma, respectively. This condition is satisfied in the G case. According to the electric field in both cases, the ratio between laser peak intensities is  , as
, as 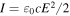 [16]. Due to the self-focusing, the peak intensity in the G case is increased by ∼10%. Thus the positron generation will be enhanced in the G case comparing with the H case. Due to the self-generated electromagnetic field, positrons generated in the G case are compressed to a higher density with a clearer wave-like density profile (figures 5(c) and (d)). It is shown that, the maximal positron density in the G case is ∼1.1nc, one order of magnitude higher than that in the H case (0.3nc). The energy density is even two orders of magnitude higher in the former. Besides, when generated in the homogeneous plasmas, positrons are pushed forward without confinement. The average positron divergence angle in the H case (32.84°) is much larger than that in the G case (23.76°).
[16]. Due to the self-focusing, the peak intensity in the G case is increased by ∼10%. Thus the positron generation will be enhanced in the G case comparing with the H case. Due to the self-generated electromagnetic field, positrons generated in the G case are compressed to a higher density with a clearer wave-like density profile (figures 5(c) and (d)). It is shown that, the maximal positron density in the G case is ∼1.1nc, one order of magnitude higher than that in the H case (0.3nc). The energy density is even two orders of magnitude higher in the former. Besides, when generated in the homogeneous plasmas, positrons are pushed forward without confinement. The average positron divergence angle in the H case (32.84°) is much larger than that in the G case (23.76°).
Figure 5. Distributions of the transverse laser electric field (V m−1) in simulations of the density gradient-distribution (G) target (a) and the density homogeneous-distribution (H) target (b) at t = 20T0 with a = 700. Density distributions of positrons in unit of nc at t = 20T0 in simulations of the G case (c) and the H case (d) with a = 700.
Download figure:
Standard image High-resolution imageDifferences in spectra are also presented in figure 6. In the G case, photons are radiated both via the Compton back-scattering process and the electron oscillation. However, in the H case, when the ultra intense laser pulse irradiates the target, electrons are all expelled without a bubble being generated behind. Photons are only radiated via the Compton back-scattering of electrons, which overcome the ponderomotive force and run into the laser from the pulse front [17]. Besides, the laser self-focusing is not initiated in the H case. Thus, on the one hand, much more photons are radiated in the G case. On the other hand, the photon cutoff energy (∼16 GeV) is much higher than ∼7GeV in the H case (figure 6(a)). Finally, more positrons are generated in the G case. The ratio of positron yields in both cases is 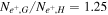 . It is also noted from figure 6(b) that the positron energy (>20 GeV) in the G case is much higher than that in the H case. In the G case, positrons are compressed with a wave-like density profile, and accelerated via the DLA (figure 5(c)). However, in the H case, positrons are pushed forwardly by the laser ponderomotive force without transverse collimation (figure 5(d)).
. It is also noted from figure 6(b) that the positron energy (>20 GeV) in the G case is much higher than that in the H case. In the G case, positrons are compressed with a wave-like density profile, and accelerated via the DLA (figure 5(c)). However, in the H case, positrons are pushed forwardly by the laser ponderomotive force without transverse collimation (figure 5(d)).
Figure 6. Spectrum of photons (a) and positrons (b) at t = 20T0 in simulations of the G case and the H case with a = 700.
Download figure:
Standard image High-resolution imageThe laser intensity plays a key role in positron generation and acceleration. A parameter scan of the normalized laser amplitude is conducted with all the other parameters unchanged. When the laser is ultra intense (e.g. a = 900), the generated pairs will further radiate photons to join in the BW process. On the one hand, a cascade is induced with more positrons generated. On the other hand, the generated positrons are well compressed as a result of the RRT and the self-generated electromagnetic field. As the laser propagates in the plasma channel, the positron maximal density is consistently increased to ∼9.1nc (figure 7(a)). It is noted from figure 7(b) that increasing the laser intensity is an effective way to raise the positron maximal density. In the positron acceleration, an ultra intense laser can induce more positrons generated, and further accelerate them to higher energy (figure 7(c)). Figure 7(d) shows the positron cutoff energy as a function of the normalized laser amplitude. As the laser gets intense, the positron cutoff energy, as well as the effective temperature, directly increases with Ecutoff ∝ a. In the scaling of the DLA given by Pukhov et al [29], the electron effective temperature and the peak laser intensity have a relationship of 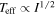 . The positron acceleration in our scheme agrees well with their theory, which confirms the DLA of positrons. However, it is difficult to obtain an intensity above 1023 W cm−2 with state-of-art lasers. Actually, when the normalized laser amplitude is only 70 in our scheme, there are still copious positrons (∼9055e+) generated with a maximal density >0.01nc and a conversion efficiency of ∼8.3 × 10−10 from laser energy to positrons.
. The positron acceleration in our scheme agrees well with their theory, which confirms the DLA of positrons. However, it is difficult to obtain an intensity above 1023 W cm−2 with state-of-art lasers. Actually, when the normalized laser amplitude is only 70 in our scheme, there are still copious positrons (∼9055e+) generated with a maximal density >0.01nc and a conversion efficiency of ∼8.3 × 10−10 from laser energy to positrons.
Figure 7. Evolutions of the positron density in simulations with different laser intensities (a). The positron density (b) and its cutoff energy (d) at t = 28T0 as a function of the normalized laser amplitude. Positron spectra at t = 28T0 in simulations with different laser intensities (c).
Download figure:
Standard image High-resolution image5. Conclusions
To conclude, we propose a compact scheme encompassing the positron generation, compression and acceleration. When an ultra intense laser irradiates a plasma channel, a bubble-like channel is formed behind. Due to the special transverse density distribution, the laser self-focusing is induced to increase the laser intensity. When the charge separation field is greater than the field due to laser ponderomotive potential, electrons in front of the pulse enter into the laser field, and radiate photons via the Compton back-scattering process. The expelled electrons run backwards along the bubble inner wall, and then they are injected into the bubble from the tail. These electrons radiate photons due to the transverse oscillation. All the radiated photons from both processes further interact with the laser photons to generate copious positrons via the BW process. When generated, positrons are compressed into a wave-like density profile due to the self-generated electromagnetic field and the RRT. They are accelerated to high-energy via the DLA. Both the radiation process and the positron acceleration process in gradient-distribution plasmas and uniform plasmas are different. Besides, the laser self-focusing is initiated in our scheme. Thus, both the positron maximal density and the energy in our scheme are much higher than that in uniform plasmas. The laser intensity plays a crucial role in increasing the positron density and energy. The relationship between the laser intensity and the positron energy in our scheme agrees well with the DLA theory. The compact scheme can facilitate the high-density and high-energy positron beam generation in labs and its further applications in laboratory astrophysics, particle physics, etc.
Acknowledgments
This work is financially supported by the National Key Research and Development Program of China (Grant No. 2018YFA0404802), the National Natural Science Foundation (Grant Nos. 11805278, 11875319, 11875091, 11675264 and 61602506), the Hunan Provincial Natural Science Foundation of China (Grant No. 2017JJ1003), the Hubei Provincial Natural Science Foundation of China (Grant No. 2016CFB307), and the Research Project of NUDT (ZK18-02-02). The authors wish to acknowledge CFSA at University of Warwick for allowing usage of EPOCH.